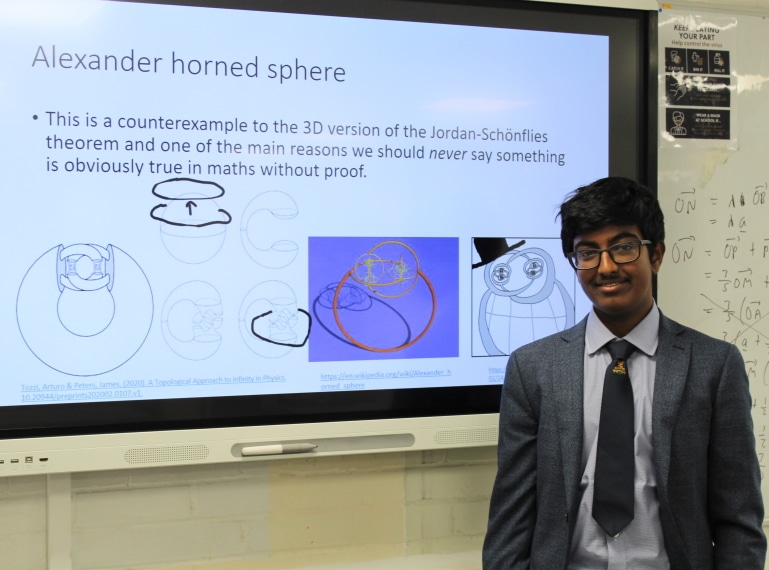
Year 12’s Shankar Vallinayagam took his place on the stage alongside professional mathematicians as a speaker at the 2022 Maths Fest.
Shankar was among 45 Year 12 mathematicians from QE to attend the annual series of lectures at The Royal Institution in London.
He was selected as a speaker after his video submission for a related Mathematics competition – the Maths Slam – was picked as one of the winning entries by the judges. He was one of four winners who gave presentations on the day.
Mathematics teacher Kirtan Shah said: “I know that many of our boys not only enjoyed the day, but also really relished the opportunity to learn about fascinating aspects of maths and its applications in the real world. As one of our students, Haipei Jiang, put it afterwards ‘It was great to be in an environment where so many other students appreciated really cool maths.’”
As in previous years, the day was chaired by Mathematics YouTuber and ‘stand up mathematician’ Matt Parker.
The day began with Professor Jennifer Rogers, Vice President for Statistical Research and Consultancy at PHASTAR, the London-based international biometrics contract research organisation, giving a talk on Stats to Save the World. As the lead statistician on a treatment trial for Covid-19, she explained the importance of sample size for clinical trials and the factors which help to determine how large a sample size should be.
Next was internet mathematician and public speaker James Grime, who talked about hidden Mathematics behind the digital world, from looking at how wifi signals are communicated to how cryptanalysis was used to break codes from Germany’s Lorenz cipher machines during World War II.
Host Matt Parker explained that our brains naturally think of numbers using a logarithmic scale, yet the modern world uses a linear system of numbers – which can easily confuse people in understanding how big large numbers actually are.
Puzzle expert and author Rob Eastaway looked at ‘fairness’ and ‘guilt’, telling the audience that chimpanzees have been seen to grasp the concept of fairness, refusing a treat if they felt they were receiving preferential treatment over their fellow chimps.
Mathematics teacher and examinations expert Nicole Cozens shared her top five tips for exam success from her experience of marking papers for 15 years, starting with: Always quote the formula first when using it in a question – this is to show the examiner that you know the formula, even if you end up making a mistake in how you use it.
Ben Sparks, musician and star of the educational YouTube channel, Numberphile, ended the day by explaining the Mathematics of the notes in an octave and sharing how sine waves, trigonometry and complex numbers are used to make noise-cancelling headphones work.
Shankar’s short talk was entitled The Alexander Horned Sphere: he came across the sphere, an object found in the branch of Mathematics known as topology, during his research for his Extended Project Qualification (EPQ) dissertation. Mr Shah said: “His talk interested the audience and got them thinking about why we shouldn’t say something is ‘obviously true’ in maths without proof.”
During breaks, the boys visited the event’s Maths Village, where they could enjoy mini-mathematical activities and meet people who use Mathematics every day at university and in commerce.
The boys were accompanied by Mr Shah and his fellow Mathematics teachers, Deljoo Mahdmina and Heena Haq.
Reflecting on the Maths Fest afterwards, pupil Rajveer Mukherjee said: “I particularly enjoyed it as although the talks were incredibly interesting, they also proved to be accessible to all, while leaving room for further research into the topics.” For his part, Abir Mohammed loved the opportunity to meet “renowned maths celebrities”.
Dr Bhattacharya’s book, entitled The Man from the Future: The Visionary Life of John von Neumann, was named a Financial Times and Times Literary Supplement Book of the Year in 2021.
In his talk, Dr Bhattacharya mentioned the Manhattan Project as well as, inter alia, von Neumann’s contributions to set theory, game theory and the development of the first programmable digital computer.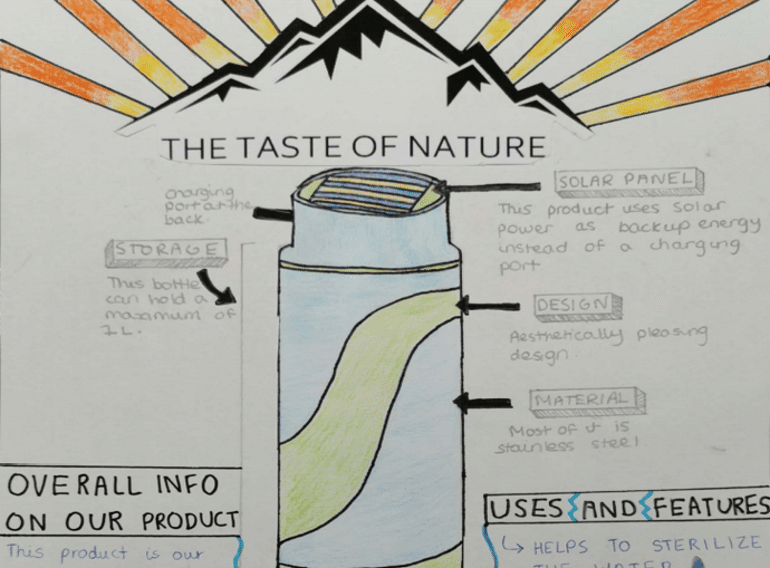
Team 4’s Water UVC bottle could thus benefit many millions across the developing world without access to safe drinking water, the boys explained in their richly illustrated, 31-page PowerPoint presentation. They even included an option for the UV lamp to be solar-powered to make the bottle viable for people who could not afford mains electricity.
Making the announcement that Team 4 had won, Assistant Head (Pupil Progress) Sarah Westcott said: “During last term’s lockdown, our usual face-to-face careers activities for Year 9 in this important period of their School careers had to be reimagined. We amended our plans so that boys could work from home, while still developing important work-related skills such as creativity, teamwork, independence and the ability to communicate their ideas.”
Question: Two congruent pentagons are each formed by removing a right-angled isosceles triangle from a square of side-length 1.
In a special lecture delivered via Zoom, Chris Budd, Professor of Applied Mathematics at Bath, first explained to the whole year group what mathematical modelling is, with contributions also coming from a number of his PhD students. In a highly illustrated presentation, he then set out modelling’s crucial role in determining the best strategy for fighting the pandemic, even drilling down into issues such as how shopping can be made safer in a pandemic.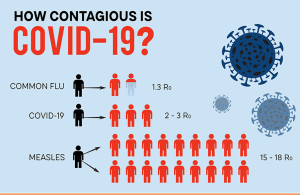
The professor started his talk by revealing that, although not an Elizabethan, he had been born in Friern Barnet and had moved to Harrow Weald at primary school age, before going up to Cambridge to read Mathematics.
Professor Budd went on to explain how modelling has been used in the case of the Covid pandemic. There are three basic questions, he said. Firstly, how will the epidemic grow if the authorities do nothing (which was the case for the 1918 Spanish ‘flu epidemic)? Secondly, how can we stop the number of cases growing? And third, how should we change our behaviour to keep safe?
Modifying behaviour patterns is simply the most effective way to prevent the spread of Covid, which is why wearing masks, staying in closed bubbles and keeping 2m away from other people are the strategies the Government has been promoting most, he said.
Congratulating him, QE’s Head of Technology Michael Noonan said: “Ashwin is an outstanding Technology student who has had a tremendous year. Despite the challenges of the school closure, or perhaps even taking advantage of them, he threw himself into many competitions using his vast technological experience. He was successful in eight competitions on a local, national and, with his latest win, international level. He should be extremely proud of his achievements this year, and he undoubtedly has a bright future ahead of him!”